题目内容
已知函数 ,x∈R.
,x∈R.
(1)写出函数的单调减区间、对称轴方程和对称中心;
(2)当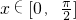 时,求y的取值范围;
时,求y的取值范围;
(3)说明由y=sinx的图象经过怎样的变换可以得到函数 的图象.
的图象.
解:(1)由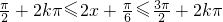 ,k∈Z
,k∈Z
得函数的单调减区间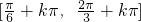 .k∈Z.
.k∈Z.
由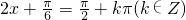 ,得对称轴方程
,得对称轴方程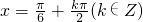
由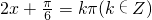 ,得对称中心
,得对称中心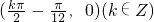
(2)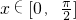 ,得
,得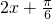
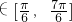 ,
, ,y∈[-1.2].
,y∈[-1.2].
(3)函数 的图象可以由y=sinx的图象先向左平移
的图象可以由y=sinx的图象先向左平移 个单位,
个单位,
再将所有点的横坐标变为原来的 (纵坐标不变),
(纵坐标不变),
最后将所有点的纵坐标变为原来的 (横坐标不变)而得到.
(横坐标不变)而得到.
分析:(1)利用正弦函数的单调减区间求出函数的单调减区间,正弦函数的对称轴方程与对称中心坐标求出函数的对称轴方程和对称中心;
(2)当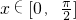 时,求出
时,求出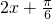 的范围,然后求y的取值范围;
的范围,然后求y的取值范围;
(3)通过左加右减以及伸缩变换的原则,由y=sinx的图象经过向左平移 个单位,横坐标变为原来的
个单位,横坐标变为原来的 ,然后将所有点的纵坐标变为原来的
,然后将所有点的纵坐标变为原来的 得到函数
得到函数 的图象.
的图象.
点评:本题考查考查正弦函数的单调性,对称中心与对称轴方程的求法,三角函数的值与图象的变换,考查基本知识的掌握情况,考查计算能力.
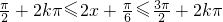 ,k∈Z
,k∈Z得函数的单调减区间
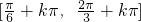 .k∈Z.
.k∈Z.由
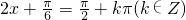 ,得对称轴方程
,得对称轴方程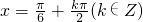
由
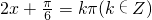 ,得对称中心
,得对称中心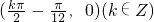
(2)
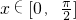 ,得
,得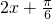
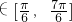 ,
, ,y∈[-1.2].
,y∈[-1.2].(3)函数
 的图象可以由y=sinx的图象先向左平移
的图象可以由y=sinx的图象先向左平移 个单位,
个单位,再将所有点的横坐标变为原来的
 (纵坐标不变),
(纵坐标不变),最后将所有点的纵坐标变为原来的
 (横坐标不变)而得到.
(横坐标不变)而得到.分析:(1)利用正弦函数的单调减区间求出函数的单调减区间,正弦函数的对称轴方程与对称中心坐标求出函数的对称轴方程和对称中心;
(2)当
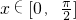 时,求出
时,求出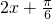 的范围,然后求y的取值范围;
的范围,然后求y的取值范围;(3)通过左加右减以及伸缩变换的原则,由y=sinx的图象经过向左平移
 个单位,横坐标变为原来的
个单位,横坐标变为原来的 ,然后将所有点的纵坐标变为原来的
,然后将所有点的纵坐标变为原来的 得到函数
得到函数 的图象.
的图象.点评:本题考查考查正弦函数的单调性,对称中心与对称轴方程的求法,三角函数的值与图象的变换,考查基本知识的掌握情况,考查计算能力.

练习册系列答案
 全能练考卷系列答案
全能练考卷系列答案
相关题目
 (x∈R).若
(x∈R).若 ,
, .求cos2x的值.
.求cos2x的值. ,x∈R,且
,x∈R,且
 ,
, ,
, ,求cos(α+β)的值.
,求cos(α+β)的值.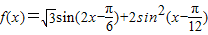 (x∈R).
(x∈R). ,x∈R
,x∈R
 ,x∈R,且
,x∈R,且
 ,
, ,
, ,求cos(α+β)的值.
,求cos(α+β)的值.