题目内容
对于函数f(x),其定义域为D,若任取x1、x2∈D,且x1≠x2,若f(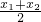 )>
)> [f(x1)+f(x2)],则称f(x)为定义域上的凸函数.
[f(x1)+f(x2)],则称f(x)为定义域上的凸函数.
(1)设f(x)=ax2(a>0),试判断f(x)是否为其定义域上的凸函数,并说明原因;
(2)若函数f(x)=㏒ax(a>0,且a≠1)为其定义域上的凸函数,试求出实数a的取值范围.
解:(1)f(x)不是其定义域上的凸函数.
f(x)的定义域为R,设x1≠x2,则
f(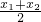 )-
)- [f(x1)+f(x2)]=a
[f(x1)+f(x2)]=a -
- (a
(a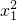 -a
-a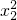 )=-
)=-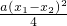 <0,
<0,
∴f(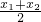 )<
)< [f(x1)+f(x2)],
[f(x1)+f(x2)],
∴f(x)不是其定义域上的凸函数
(2)∵f(x)的定义域为(0,+∞),且f(x)在(0,+∞)内是凸函数,
∴f(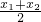 )>
)> [f(x1)+f(x2)],
[f(x1)+f(x2)],
即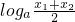 >
> (logax1+logax2)=
(logax1+logax2)=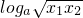 ①
①
∵x1、x2∈(0,+∞),且x1≠x2,
∴ -x1x2=
-x1x2= >0,即
>0,即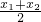 >
>
故要①成立,则a>1.
∴实数a的取值范围是(1,+∞)
分析:(1)根据凸函数的定义,作差f(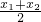 )-
)- [f(x1)+f(x2)]判断即可;
[f(x1)+f(x2)]判断即可;
(2)依题意,f(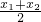 )>
)> [f(x1)+f(x2)]?
[f(x1)+f(x2)]?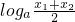 >
>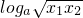 ,通过比较其真数的大小即可求得实数a的取值范围.
,通过比较其真数的大小即可求得实数a的取值范围.
点评:本题考查对数函数的单调性,考查作差法,着重考查推理证明的逻辑思维能力,属于难题.
f(x)的定义域为R,设x1≠x2,则
f(
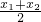 )-
)- [f(x1)+f(x2)]=a
[f(x1)+f(x2)]=a -
- (a
(a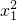 -a
-a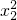 )=-
)=-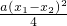 <0,
<0,∴f(
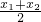 )<
)< [f(x1)+f(x2)],
[f(x1)+f(x2)],∴f(x)不是其定义域上的凸函数
(2)∵f(x)的定义域为(0,+∞),且f(x)在(0,+∞)内是凸函数,
∴f(
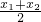 )>
)> [f(x1)+f(x2)],
[f(x1)+f(x2)],即
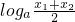 >
> (logax1+logax2)=
(logax1+logax2)=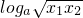 ①
①∵x1、x2∈(0,+∞),且x1≠x2,
∴
 -x1x2=
-x1x2= >0,即
>0,即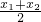 >
>
故要①成立,则a>1.
∴实数a的取值范围是(1,+∞)
分析:(1)根据凸函数的定义,作差f(
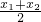 )-
)- [f(x1)+f(x2)]判断即可;
[f(x1)+f(x2)]判断即可;(2)依题意,f(
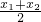 )>
)> [f(x1)+f(x2)]?
[f(x1)+f(x2)]?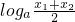 >
>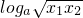 ,通过比较其真数的大小即可求得实数a的取值范围.
,通过比较其真数的大小即可求得实数a的取值范围.点评:本题考查对数函数的单调性,考查作差法,着重考查推理证明的逻辑思维能力,属于难题.

练习册系列答案
相关题目
 )>
)> [f(x1)+f(x2)],则称f(x)为定义域上的凸函数.
[f(x1)+f(x2)],则称f(x)为定义域上的凸函数.