题目内容
椭圆C的方程为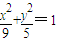 ,F1、F2分别为C的左、右焦点,点A的坐标为(1,1),P是C上的任意一点,给出下列结论:
,F1、F2分别为C的左、右焦点,点A的坐标为(1,1),P是C上的任意一点,给出下列结论:①|PF1|-|PF2|有最大值5,②|PF1|•|PF2|有最大值9,③|PF1|2+|PF2|2有最大值18,④|PF1|+|PA|有最小值
 ,其中正确结论的序号是 .
,其中正确结论的序号是 .
【答案】分析:①利用三角形两边之差小于第三边可证明当点P在x轴上时,|PF1|-|PF2|有最大值2c,由椭圆标准方程计算焦距即可;
②利用椭圆定义知|PF1|+|PF2|为定值2a,再利用均值定理求积|PF1|•|PF2|的最大值即可;
③利用焦半径公式设P点横坐标为x,则|PF1|2+|PF2|2可转化为关于x0的一元函数,由x的范围即可求得|PF1|2+|PF2|2的最大值;
④由椭圆的定义结合三角形的性质,即可判断
解答:解:①当P点不在x轴上时,P,F1,F2,三点构成三角形,此时|PF1|-|PF2|<|F1F2|,
∵|F1F2|=4,∴|PF1|-|PF2|<4,
当P点在x轴上时,|PF1|-|PF2|=|F1F2|=4,∴|PF1|-|PF2|≤4,即①|PF1|-|PF2|有最大值4,①错误.
②∵P点在椭圆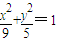 上,∴|PF1|+|PF2|=|2a=6,
上,∴|PF1|+|PF2|=|2a=6,
∵|PF1|>0,|PF2|>0,∴|PF1|•|PF2|≤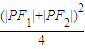 =9,∴|PF1|•|PF2|有最大值9,②正确.
=9,∴|PF1|•|PF2|有最大值9,②正确.
③根据椭圆方程,可得椭圆的离心率为
设P点横坐标为x,则|PF1|=a+ex,|PF2|=a-ex,
∴|PF1|2+|PF2|2=(a+ex)2+(a-ex)2=2a2+2e2x2=18+ x2
x2
∵P点在椭圆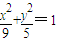 上,∴x2≤9,∴18+
上,∴x2≤9,∴18+ x2≤26,∴PF1|2+|PF2|2有最大值26,
x2≤26,∴PF1|2+|PF2|2有最大值26,
∴③错误,
④由椭圆的定义可知|PF1|+|PF2|=2a,,|PF1|+|PA|+|F2A|≥|PF1|+|PF2|
∴|PF1|+|PA|≥|PF1|+|PF2|-|F2A|=6- ,所以有最小值
,所以有最小值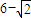 ,正确.
,正确.
故答案为:②④.
点评:本题考查了椭圆的标准方程的意义,椭圆定义的应用,椭圆的几何性质,利用均值定理和函数求最值的方法.
②利用椭圆定义知|PF1|+|PF2|为定值2a,再利用均值定理求积|PF1|•|PF2|的最大值即可;
③利用焦半径公式设P点横坐标为x,则|PF1|2+|PF2|2可转化为关于x0的一元函数,由x的范围即可求得|PF1|2+|PF2|2的最大值;
④由椭圆的定义结合三角形的性质,即可判断
解答:解:①当P点不在x轴上时,P,F1,F2,三点构成三角形,此时|PF1|-|PF2|<|F1F2|,
∵|F1F2|=4,∴|PF1|-|PF2|<4,
当P点在x轴上时,|PF1|-|PF2|=|F1F2|=4,∴|PF1|-|PF2|≤4,即①|PF1|-|PF2|有最大值4,①错误.
②∵P点在椭圆
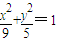 上,∴|PF1|+|PF2|=|2a=6,
上,∴|PF1|+|PF2|=|2a=6,∵|PF1|>0,|PF2|>0,∴|PF1|•|PF2|≤
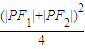 =9,∴|PF1|•|PF2|有最大值9,②正确.
=9,∴|PF1|•|PF2|有最大值9,②正确.③根据椭圆方程,可得椭圆的离心率为

设P点横坐标为x,则|PF1|=a+ex,|PF2|=a-ex,
∴|PF1|2+|PF2|2=(a+ex)2+(a-ex)2=2a2+2e2x2=18+
 x2
x2∵P点在椭圆
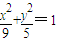 上,∴x2≤9,∴18+
上,∴x2≤9,∴18+ x2≤26,∴PF1|2+|PF2|2有最大值26,
x2≤26,∴PF1|2+|PF2|2有最大值26,∴③错误,
④由椭圆的定义可知|PF1|+|PF2|=2a,,|PF1|+|PA|+|F2A|≥|PF1|+|PF2|
∴|PF1|+|PA|≥|PF1|+|PF2|-|F2A|=6-
 ,所以有最小值
,所以有最小值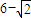 ,正确.
,正确.故答案为:②④.
点评:本题考查了椭圆的标准方程的意义,椭圆定义的应用,椭圆的几何性质,利用均值定理和函数求最值的方法.

练习册系列答案
相关题目
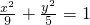 ,F1、F2分别为C的左、右焦点,点A的坐标为(1,1),P是C上的任意一点,给出下列结论:
,F1、F2分别为C的左、右焦点,点A的坐标为(1,1),P是C上的任意一点,给出下列结论: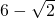 ,其中正确结论的序号是________.
,其中正确结论的序号是________.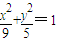 ,F1、F2分别为C的左、右焦点,P是C上的任意一点,给出下列结论:
,F1、F2分别为C的左、右焦点,P是C上的任意一点,给出下列结论: