题目内容
过点P(1，0)的直线l与曲线C：
+y2=1交于A、B两点,过点P还有一直线l′与曲线C交于C、D两点(与A、B不重合),若A、C、B、D四点共圆,试求l与l′的倾斜角之间应满足什么条件？并说明理由.
解析：应满足倾斜角互补.
由题设可知l′:(t为参数)，θ∈［0,π)，
则(1+t cosθ)2+2t2 sin2θ-2=0，
即(1+sin2θ)t2+2t cosθ-1=0.
∴|PD|·|PC|=|t1 ·t2|=
.
而|PA|·|PB|=|t1·t2|=
,
∵A、C、B、D四点共圆,
∴|PA|·|PB|=|PC|·|PD|,
即
=
.
∴sin2α=sin2θ.
∴sinα=sinθ.
∵A、B、C、D不重合,
故α+θ=π,即两直线倾斜角互补.
练习册系列答案
华东师大版一课一练系列答案
相关题目
过点A（a,0）,B(0,b)的直
，原点到该直线的距离为
.
求直线MN的方程；
交椭圆于P、Q两点，以PQ为直径的圆过点D（1，0）？若存在，求出k的值；若不存在，请说明理由。
已知抛物线C的顶点在原点, 焦点为F(0, 1).
=1（a＞b＞0）离心率为
，且过P（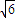
，
）．
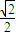，0），且与开口朝上，顶点在原点的抛物线C切于第二象限的一点N，直 线l与椭圆E交于A，B两点，与y轴交与D点，若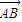
=
，
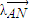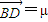
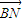，且λ+μ=
，求抛物线C的标准方程．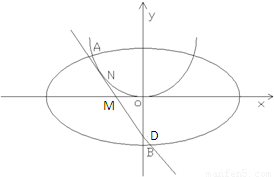
=1（a＞b＞0）离心率为
，且过P（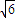
，
）．
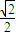，0），且与开口朝上，顶点在原点的抛物线C切于第二象限的一点N，直 线l与椭圆E交于A，B两点，与y轴交与D点，若
=
，
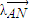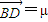
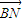，且λ+μ=
，求抛物线C的标准方程．