题目内容
当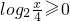
，时，求函数
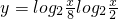的值域．
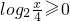解：由可得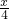 
≥1，故有x≥4，log2x≥2．
函数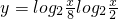=（log2x-3）（log2x-1）．
令t=log2x≥2，则函数y=（t-3）（t-1）在[2，+∞）上是增函数，
故当t=2时，函数y=（t-3）（t-1）取得最小值为-1，
故函数的值域为[-1，+∞）．
分析：由条件可得log2x≥2，令t=log2x≥2，则函数y=（t-3）（t-1）在[2，+∞）上是增函数，再利用函数的单调性求出函数的值域．
点评：本题主要考查对数函数的单调性和特殊点，二次函数的性质的应用，属于中档题．
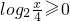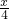可得 ≥1，故有x≥4，log2x≥2．函数
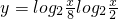=（log2x-3）（log2x-1）．令t=log2x≥2，则函数y=（t-3）（t-1）在[2，+∞）上是增函数，
故当t=2时，函数y=（t-3）（t-1）取得最小值为-1，
故函数的值域为[-1，+∞）．
分析：由条件可得log2x≥2，令t=log2x≥2，则函数y=（t-3）（t-1）在[2，+∞）上是增函数，再利用函数的单调性求出函数的值域．
点评：本题主要考查对数函数的单调性和特殊点，二次函数的性质的应用，属于中档题．
练习册系列答案
教学练新同步练习系列答案
课前课后同步练习系列答案
相关题目
的值；（2）设
的三边a、b、c满足
，且边b所对的角
的取值集合为M. 当
M时，求函数
的值域.
是不为零的常数，二次函数
的定义域为R，函数
为偶函数． 函数
的定义域为
．
、
时，求函数
的值域；
、
，使函数
？如果存在，求出
，时，求函数
的值域．
，时，求函数
的值域．