题目内容
已知α,β均为锐角,且cosα=
,tan(α-β)=-
.
(1)求cos(α-β)的值;
(2)求sinβ的值.
| 4 |
| 5 |
| 1 |
| 3 |
(1)求cos(α-β)的值;
(2)求sinβ的值.
(1)∵0<α<
,cosα=
,∴sinα=
=
,∴tanα=
=
.
∵tan(α-β)=
=
=-
,解得tanβ=
.
联立
,解得
.
∴cos(α-β)=cosαcosβ+sinαsinβ=
×
+
×
=
.
(2)由(1)可得sinβ=
.
| π |
| 2 |
| 4 |
| 5 |
| 1-cos2α |
| 3 |
| 5 |
| sinα |
| cosα |
| 3 |
| 4 |
∵tan(α-β)=
| tanα-tanβ |
| 1+tanαtanβ |
| ||
1+
|
| 1 |
| 3 |
| 13 |
| 9 |
联立
|
|
∴cos(α-β)=cosαcosβ+sinαsinβ=
| 4 |
| 5 |
9
| ||
| 50 |
| 3 |
| 5 |
13
| ||
| 50 |
3
| ||
| 10 |
(2)由(1)可得sinβ=
13
| ||
| 50 |

练习册系列答案
相关题目
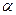 +
+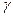 )=sin2
)=sin2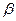 ”是“
”是“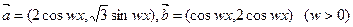 ,函数
,函数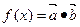 的最小正周期为
的最小正周期为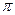 : (Ⅰ) 求
: (Ⅰ) 求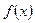 的单调增区间 (Ⅱ) 在
的单调增区间 (Ⅱ) 在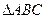 中,
中,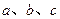 分别是角A、B、C的对边,若
分别是角A、B、C的对边,若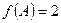 ,
,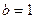 ,
, 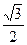 ,求
,求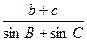 的值
的值 的最小值是____________________ .
的最小值是____________________ .
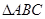 中,角
中,角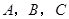 所对的边分别为
所对的边分别为 ,角
,角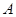 为锐角,且
为锐角,且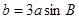 ,则
,则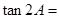 .
.