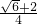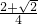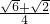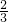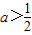题目内容
设对任意实数x＞0，y＞0，若不等式x+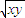
≤a（x+2y）恒成立，则实数a的最小值为（ ）A．
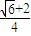
B．
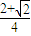
C．
D．
【答案】分析：分离参数可得：a≥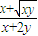
=
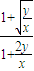，令
，则a≥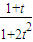
令1+t=m（m＞1），
=
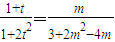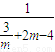，求出最大值，即可求得a的最小值．
解答：解：分离参数可得：a≥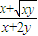
=
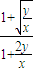
令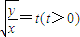
，则a≥
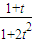
令1+t=m（m＞1），
=
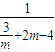
∵m＞1，∴
（当且仅当m=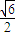
时，取等号）
∴
∴
∴
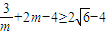
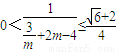
∴a≥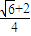
∴a的最小值为
．
点评：本题考查恒成立问题，考查基本不等式的运用，解题的关键是分离参数，转化为求函数的最值．
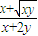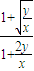=，令，则a≥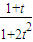令1+t=m（m＞1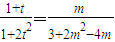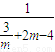），=，求出最大值，即可求得a的最小值．解答：解：分离参数可得：a≥
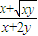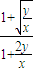
=令
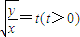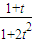
，则a≥令1+t=m（m＞1），
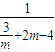
=∵m＞1，∴
（当且仅当m=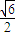时，取等号）∴
∴
∴
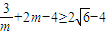
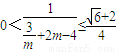
∴a≥
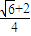
∴a的最小值为
．点评：本题考查恒成立问题，考查基本不等式的运用，解题的关键是分离参数，转化为求函数的最值．
练习册系列答案
相关题目
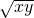≤a（x+2y）恒成立，则实数a的最小值为
