题目内容
若 是关于
是关于 的一元二次方程
的一元二次方程 的两个根,则方程的两个根
的两个根,则方程的两个根 和系数
和系数 有如下关系:
有如下关系: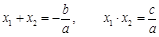 . 我们把它们称为根与系数关系定理. 如果设二次函数
. 我们把它们称为根与系数关系定理. 如果设二次函数 的图象与x轴的两个交点为
的图象与x轴的两个交点为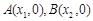 .利用根与系数关系定理我们又可以得到A、B两个交点间的距离为:
.利用根与系数关系定理我们又可以得到A、B两个交点间的距离为: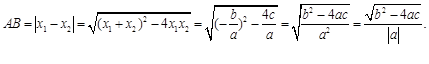
请你参考以上定理和结论,解答下列问题:
设二次函数
 的图象与x轴的两个交点为
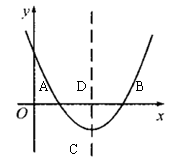
的图象与x轴的两个交点为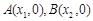 ,抛物线的顶点为
,抛物线的顶点为 ,显然
,显然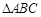 为等腰三角形.
为等腰三角形.(1)当
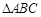 为等腰直角三角形时,求
为等腰直角三角形时,求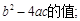
(2)当
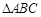 为等边三角形时,求
为等边三角形时,求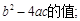
(1)4(2)12解析:
⑴ 解:当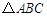 为等腰直角三角形时,过
为等腰直角三角形时,过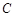 作
作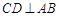 ,垂足为
,垂足为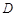 ,
,
则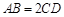 ……2分
……2分
∵抛物线与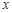 轴有两个交点,∴
轴有两个交点,∴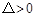 ,
,
∴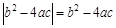 ……4分
……4分
∵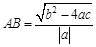
又∵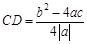 ,
,
∵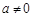 ,
,
∴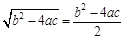 ……6分
……6分
∴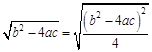
∴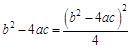
∴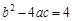 ……9分
……9分
⑵当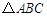 为等边三角形时,由(1)可知
为等边三角形时,由(1)可知
CD=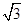 AB……10分
AB……10分
∴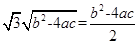 ……11分
……11分
∴b2-4ac=12……12分
(1)由于抛物线与x轴有两个不同的交点,所以b2-4ac>0;套用材料中的公式可求得线段AB的表达式,利用公式法可得到顶点C的纵坐标,进而求得斜边AB上的高(设为CD),若△ABC为等腰直角三角形,那么AB=2CD,可根据这个等量关系求出b2-4ac的值.
(2)方法同(1),只不过AB、CD的等量关系为: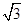 AB=2CD.
AB=2CD.
⑴ 解:当
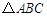 为等腰直角三角形时,过
为等腰直角三角形时,过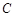 作
作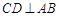 ,垂足为
,垂足为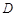 ,
,
则
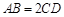 ……2分
……2分 ∵抛物线与
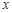 轴有两个交点,∴
轴有两个交点,∴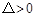 ,
,∴
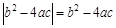 ……4分
……4分 ∵
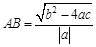
又∵
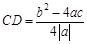 ,
, ∵
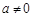 ,
,∴
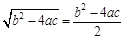 ……6分
……6分 ∴
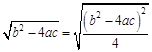
∴
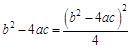
∴
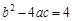 ……9分
……9分 ⑵当
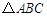 为等边三角形时,由(1)可知
为等边三角形时,由(1)可知CD=
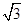 AB……10分
AB……10分∴
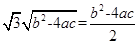 ……11分
……11分∴b2-4ac=12……12分
(1)由于抛物线与x轴有两个不同的交点,所以b2-4ac>0;套用材料中的公式可求得线段AB的表达式,利用公式法可得到顶点C的纵坐标,进而求得斜边AB上的高(设为CD),若△ABC为等腰直角三角形,那么AB=2CD,可根据这个等量关系求出b2-4ac的值.
(2)方法同(1),只不过AB、CD的等量关系为:
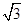 AB=2CD.
AB=2CD. 
练习册系列答案
 名校课堂系列答案
名校课堂系列答案
相关题目
若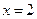 是关于
是关于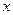 的一元二次方程
的一元二次方程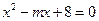 的一个根,则
的一个根,则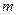 的值是( ▲ )
的值是( ▲ )
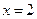 是关于
是关于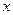 的一元二次方程
的一元二次方程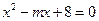 的一个根,则
的一个根,则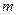 的值是( ▲ )
的值是( ▲ )A. | B. | C. | D. |
 是关于
是关于 的一元二次方程
的一元二次方程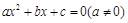 的两个根,则方程的两个根
的两个根,则方程的两个根 有如下关系:
有如下关系: . 我们把它们称为根与系数关系定理. 如果设二次函数
. 我们把它们称为根与系数关系定理. 如果设二次函数 的图象与x轴的两个交点为
的图象与x轴的两个交点为 .利用根与系数关系定理我们又可以得到A、B两个交点间的距离为:
.利用根与系数关系定理我们又可以得到A、B两个交点间的距离为:
 的图象与x轴的两个交点为
的图象与x轴的两个交点为 ,显然
,显然 为等腰三角形.
为等腰三角形.
 是关于
是关于 的一元二次方程
的一元二次方程 的一个解,则
的一个解,则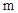 的值是 ( )
的值是 ( ) C.
5
D. 2
C.
5
D. 2 是关于
是关于 的一元二次方程
的一元二次方程 的一个解,则
的一个解,则 的值是
.
的值是
. 是关于
是关于 的一元二次方程
的一元二次方程 的一个根,则
的一个根,则 _▲_.
_▲_.