题目内容
(本小题满分12分)如图,在平面直角坐标系
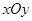 中,△ABC的A、B两个顶点在x轴上,顶点C在y轴的负半轴上.已知
中,△ABC的A、B两个顶点在x轴上,顶点C在y轴的负半轴上.已知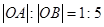 ,
, ,△ABC的面积
,△ABC的面积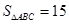 ,抛物线
,抛物线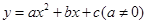
经过A、B、C三点。

【小题1】(1)求此抛物线的函数表达式;
【小题2】(2)设E是y轴右侧抛物线上异于点B的一个动点,过点E作x轴的平行线交抛物线于另一点F,过点F作FG垂直于x轴于点G,再过点E作EH垂直于x轴于点H,得到矩形EFGH.则在点E的运动过程中,当矩形EFGH为正方形时,求出该正方形的边长;
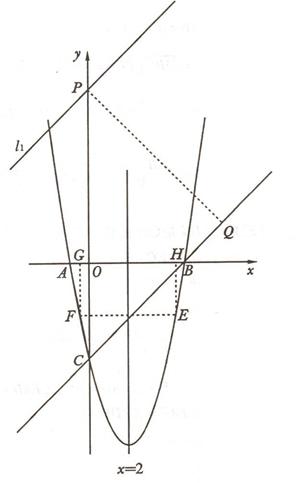
【小题3】(3)在抛物线上是否存在异于B、C的点M,使△MBC中BC边上的高为
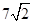 ?若存在,求出点M的坐标;若不存在,请说明理由.
?若存在,求出点M的坐标;若不存在,请说明理由.
【小题1】解:(1)∵
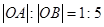 ,设
,设 ,则
,则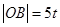
∴

又
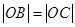 ,∴
,∴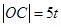
∵
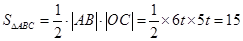
∴
 ,即
,即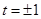 。
。而
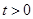 ,∴
,∴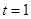 。
。∴
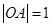 ,
,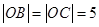
∴△ABC三个顶点的坐标分别是
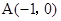 ,
, ,
,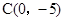
∵抛物线
 经过A、B、C三点,
经过A、B、C三点,∴设
 ,把
,把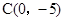 代入得
代入得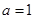
∴此抛物线的函数表达式为
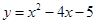
【小题2】(2)设点E的坐标为
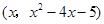 ,
,∵点E在Y轴右侧的抛物线上,∴
 。
。有抛物线的对称性,知点F与点E关于抛物线的对称轴x=2对称,
易得点F的坐标为
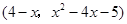 。
。要使矩形EFGH能成为正方形,有
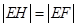 ,
,则
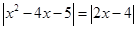
∴
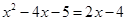 ①
①或
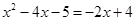 ②
②由①得,
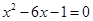 ,解得
,解得 (舍去)
(舍去)由②得,
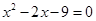 ,解得
,解得 (舍去)
(舍去)当
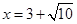 时,
时,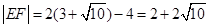
此时正方形EFGH的边长为
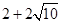 。
。当
 时,
时,
此时正方形EFGH的边长为
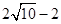 。
。∴当矩形EFGH为正方形时,该正方形的边长为
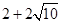 或
或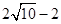
【小题3】(3)假设存在点M,使△MBC中BC边上的高为
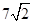 。
。∴M点应在与直线BC平行,且相距
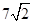 的两条平行直线
的两条平行直线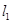 和
和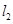 上。
上。由平行线的性质可得:
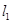 和
和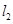 与y轴的交点到直线BC的距离也为
与y轴的交点到直线BC的距离也为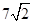 。
。如图,设
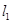 与y轴交于P点,过P作PQ与直线BC垂直,垂足为点Q,
与y轴交于P点,过P作PQ与直线BC垂直,垂足为点Q,∵
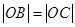 ,
,∴∠OBC=∠OCB=45°
在Rt△PQC中,
 ,∠PCQ=∠OCB=45°
,∠PCQ=∠OCB=45°∴由勾股定理,得
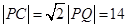
∴直线
 与y轴的交点坐标为P(0,9)
与y轴的交点坐标为P(0,9)同理可求得:
 与y轴交点坐标为
与y轴交点坐标为 ,
,易知直线BC的函数表达式
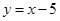 。
。∴直线
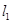 和
和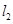 的函数表达式分别为
的函数表达式分别为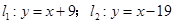 。
。根据题意,列出方程组:①
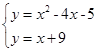 ,②
,②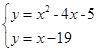
由①得,
 ,解得
,解得 ;
;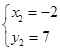
由②得,
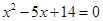
∵△="-31<0"
∴此方程无实数根。
∴在抛物线上存在点M,使△MBC中BC边上的高为
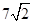 ,其坐标分别为:
,其坐标分别为:
另解:易求直线BC的表达式为:
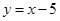
整理得
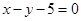
设
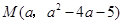
由点到直线的距离得
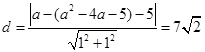
解得
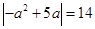
∴
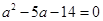 或
或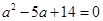 (无实数根)
(无实数根)∴
 或
或
代入得
 。解析:
。解析:略

练习册系列答案
 阅读快车系列答案
阅读快车系列答案
相关题目
 的图象经过A、B两点,根据图中信息解答下列问题:
的图象经过A、B两点,根据图中信息解答下列问题:


 同侧,在直线
同侧,在直线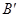 ,连接
,连接 ,与直线
,与直线


